Angle tri-section problem

Believers Information Network

One Degree
Trisecting an angle

' Therefore you cannot construct an exact
one-degree angle with ruler and compass! '
Anonymous Teacher

" And there shall be no more curse:
but the throne of God and of the Lamb shall be in it;
and his servants shall serve him: "

INTRODUCTION

Take a compass and draw a circle using any radius. Now without changing the radius, mark off as many segments as is possible on the circumference of the circle. The result will be a hexagon, a six sided polygon. The circle has been divided into six equal segments using only a compass. Now if i where to suggest to you, that we should therefore also be able to divide one of the six segments, into six equal segments, using our compass, as well as an unmarked straightedge, this should not be a daunting task. This will then enable us to divide the circle into 360 equal segments(degrees), and we will have an instrument (protractor), that we can use for the purpose of indicating direction. Your teacher will tell you this is not possible, however he is mistaken.

The purpose of this essay is to show the reader how to make a 360 degree instrument(protractor) from first principles, using only a compass and an unmarked straightedge. In order to accomplish this we will proceed to divide one of the six segments that we have already made, into six equal segments. We will then see that having done this, we are able to construct our 360 degree protractor without any difficulty.

STEP BY STEP INSTRUCTIONS TO TRISECT ANY ANGLE

STEP ONE

Using your compass draw a circle O.
Mark off one radius A1 and A2 on the right side of the circle and bisect A1A2.
Draw the symmetric axis PON.

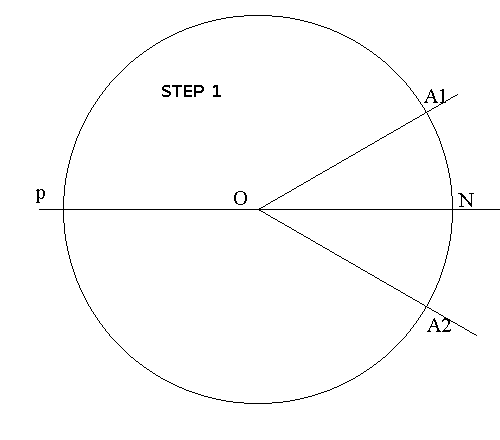
STEP TWO

Connect A1 P and A2 P.
Bisect angle P A1 O and angle P A2 O.
Q is where the bi-sections cut the axis.
With radius A1 Q draw the secondary arc A1 M A2.

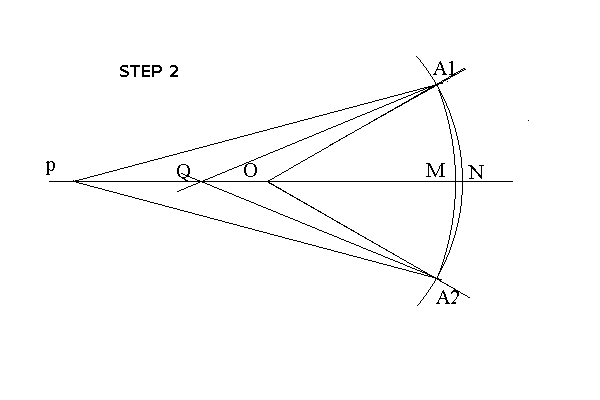
STEP THREE

Set your compass to QO and mark off MS.
With radius OS draw the primary arc B1 S B2.

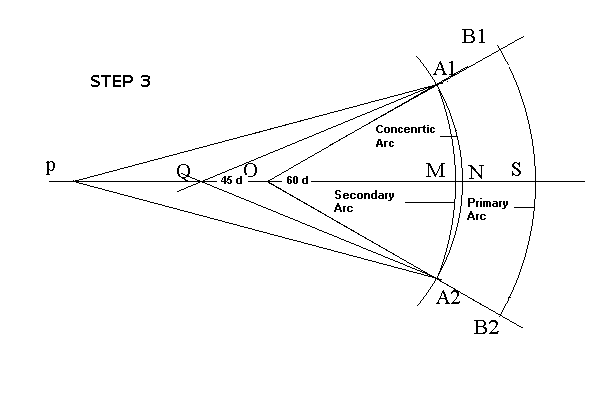
STEP FOUR

This is a very simple process and only involves a few steps.
Bi-sect B1 S1 and B2 S2 to get C1 and C2.
Use B2 C2 to divide the secondary arc at D1 and D2.
A2 D2, D2 D1, and D1 A1 are each fifteen degrees.
Connect Q and D1 , as well as Q and D2.
Connect O and D1 and extend to E1 and F1.
Connect O and D2 and extend to E2 and F2.

B2 F2, B1 F1 and F1 F2 divide the primary arc into three equal portions of twenty degrees.

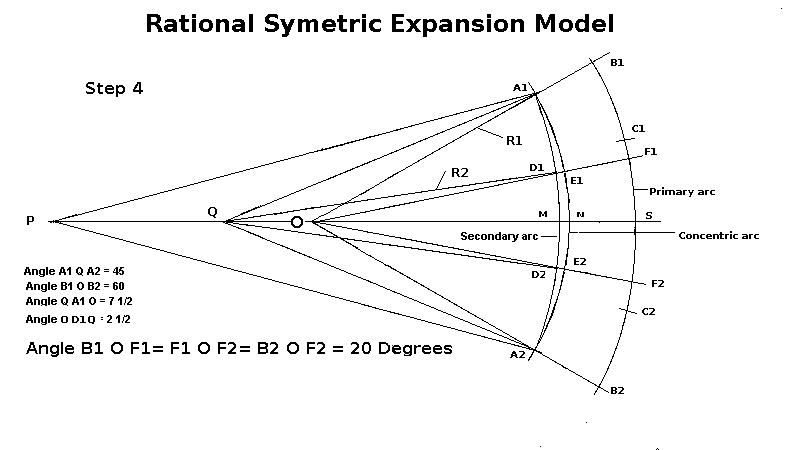
How it works

The model uses the principle of proportion to trisect a given angle.
The basic model expands a 45 degree angle by 1/3 (15 Degrees) to 60 degrees.
The model can expand any angle up to 45 degrees by one third.
The tri-section of a 60 degree angle is used in the example above.
The procedure is as follows:
Three parts(15d each) of the primary arc is transferred to the secondary arc.
Two parts of 15 degrees is then expanded by one third to 20 degrees.
I refer to the model as the rational symmetric expansion(RSE) model.

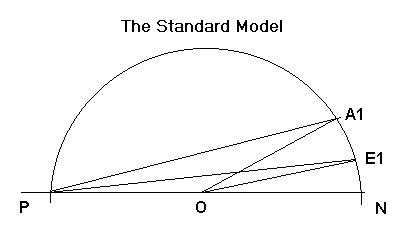
The Principle of Proportion applied (Proof)

The model uses the principle of proportion as derived from the standard model.

Let A1 O N = 30 degrees.
Then:
Angle A1 P O = 15 degrees
Angle A1 O N = 30 degrees

The ratios are 1:2

Let angle E1 O N = z degrees
Then:
Angle E1 P O = 1/2 z degrees
Angle E1 O N = z degrees

The ratios are 1:2
According to the law of proportion the relationship between the angles remains constant as E1 moves between A1 and N.
The radius O E1 and O A1 remain constant.
The sum of the angles of triangle P A1 O are 180 degrees, and the sum of the angles at O, for the straight line is also 180 degrees.

We will now use the same principles using
the revised model.

With the revised model we move point P to Q.
The constant radius is lengthened from O A1 to Q A1, and Q E2.

The relationship between angles are now as follows:

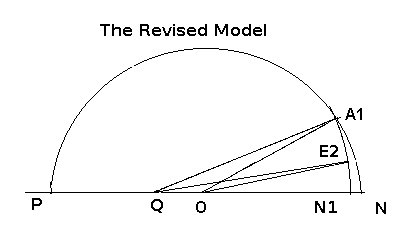
Angle Q A1 O = 7 1/2 degrees
Angle A1 Q N = 22 1/2 degrees
Angle A1 O N = 30 degrees

The ratios are 1:3:4

Let angle E2 O N be Z degrees. The relationship between the angles of spike Q E2 O will be as follows:

Angle Q E2 O = 1/4 z degrees
Angle E2 Q N = 3/4 z degrees
Angle E2 O N = z degrees

The ratios are 1:3:4

This relationship between the angles will remain constant as E2 moves between A1 and N1.
The radius Q A1 and Q E2 remain constant.

In any rational system the principle of cause and effect applies, and being a closed system nothing can be added or taken away from the system.

A RATIONAL SYSTEM OF ANGLES

The sketch below shows the relationships between all the angles of the model we used to tri-sect the 30 degree angle. To simplify the angles, one unit represents 2 1/2 degrees.

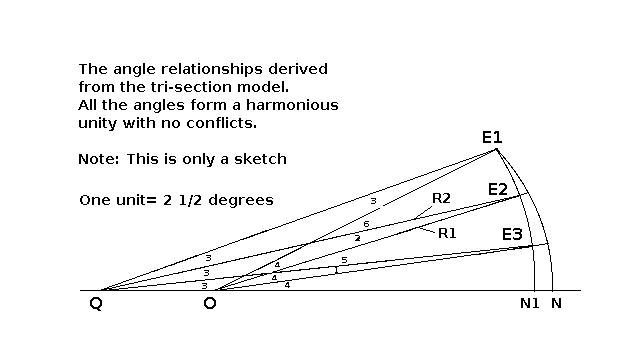
Note: All illustrations are only sketches.

ESTABLISHING THE ONE DEGREE ANGLE.

Having constructed a 10 degree angle we can now proceed with the next step.

We construct a pentagon using the same circle(radius) we used for our hexagon.
The pentagon has five equal sides of 72 degrees.
We now sub divide the 72 angle three time, giving us a 9 degrees angle.
The one degree angle is derived from the difference between the 9 and 10 degree angles.

CONCLUSION.

It is possible to construct a 360 degree protractor from first principles, using only a compass and unmarked straightedge.

For those who still have doubts, click here.