Believers Information Network



 Irrational Numbers
(Incommensurability)

" But when ye shall see the abomination of desolation,
spoken of by Daniel the prophet, standing where it
ought  not(Let him that readeth understand),
then let them that are in Judea flee to the mountains. "

The world as we know it is on the verge of a major transformation(restoration). The harvest is ripe, soon there will be a new heaven(spirit) and a new earth (perception). A paradigm shift is about to take place, a shift from an irrational world system, to a rational world system.

To illustrate my point I will refer to the present world numbering system. The present world numbering system is an extension of natural (irrational) man. It is an irrational numbering system. The abomination of desolation referred to in the book of Mark, is a symbol, it is the "ZERO". When you see it standing where the "ONE" ought to be, then you will know that the time has come. Our present numbering system is a zero base system. It will soon be replaced by a rational system, a unity base system.

Most readers will be familiar with the present numbering system, the system that is taught in schools all over the world. The world numbering system is a complex system protected by man-made rules. Rules that make no sense at all. This is why so many children struggle with math, in particular 'fractions'. When a child is told that he can multiply by zero, however he cannot divide by zero, the result is a state of confusion. The confusion is reinforced by concepts such as 'whole numbers', clearly a contradiction in terms. There can only be one whole number.

After the transformation has taken place there will be a new numbering system. The new numbering system in contrast to the present system, will be a very simple. It will consist of only two types of numbers. A whole number, which will always be "ONE", and rational numbers that form part of one. Each rational number has two parts, a numerator, and a denominator. The numerator indicates the part and the denominator indicates the whole. There will be no zero (abomination of desolation), no irrational numbers and no irrational (inconsistent) rules. All the measuring instruments will be changed, everything will be made new. A common ruler will start with one and end with one. The accuracy of the ruler will determine the number of divisions required.

Let us take a closer look at the idea of incommensurability.

One of the ideas associated with irrational numbers is the idea of incommensurability. We will now look at one of the so called 'proofs' that mathematicians dish up to unsuspecting students. This 'proof' dates back to 300 BC and is the work of  Euclid, a well respected mathematician. Let us take a look at proposition 2 of book X.

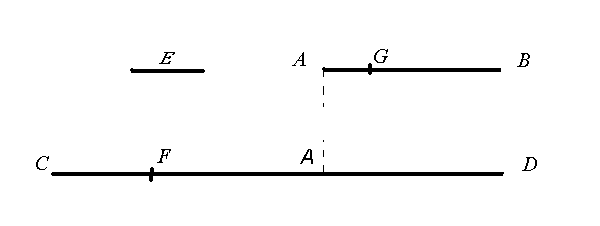
Proposition 2

If, when the less of two unequal magnitudes is continually subtracted in turn from the greater that which is left never measures the one before it, then the two magnitudes are incommensurable.

There being two unequal magnitudes AB and CD, with AB being the less, when the less is continually subtracted in turn from the greater, let that which is left over never measure the one before it.

I say that the magnitudes AB and CD are incommensurable.

If they are commensurable, then some magnitude E measures them.

Let AB, measuring FD, leave CF less than itself, let CF measuring BG, leave AG less than itself, and let this process be repeated continually, until there is left some magnitude which is less than E.

Suppose this done, and let there be left AG less than E.

Then, since E measures AB, while AB measures DF, therefore E also measures FD. But it measures the whole CD also, therefore it also measures the remainder CF.

But CF measures BG, therefore E also measures BG. But it measures the whole AB also, therefore it also measures the remainder AG, the greater the less, which is impossible.

Therefore no magnitude measures the magnitudes AB and CD. Therefore the magnitudes AB and CD are incommensurable. X.Def.1

Therefore, if, when the less of two unequal magnitudes is continually subtracted in turn from the greater that which is left never measures the one before it, then the two magnitudes are incommensurable.


Let us briefly examine Euclid's proposition.

"If, when the less of two unequal magnitudes is continually subtracted in turn from the greater, that which is left will never measures the one before it, the magnitudes will be incommensurable. "

This is indeed a very strange statement to make.
The original magnitudes consisting of the less, AD, and the greater, CA, together constitute a finite line,CD.

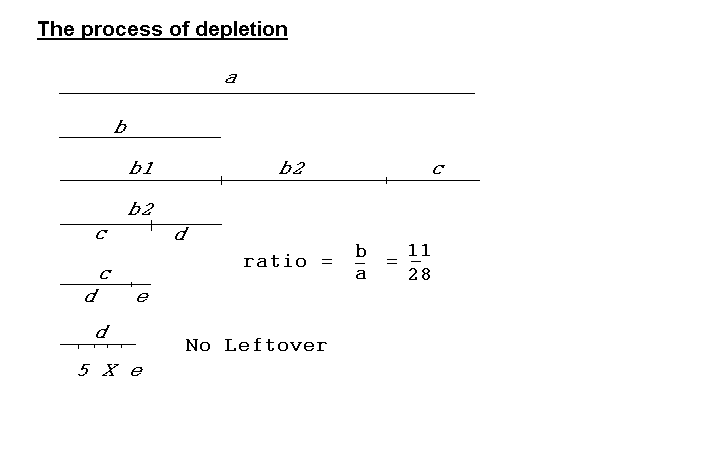
The process which Euclid describes, is a process of depletion. The basic procedure (modus operandi), is to continually subtract the less from the greater.
We can therefore expect the finite line CD to be depleted, but according to Euclid " that which is left will never measure the one before it ", which can only mean that there will always be a leftover, the finite has become infinite. The only other possibility will be that, that which is leftover, is greater than that before it, which means the process is halted prematurely thus violating the basic procedure.
So the only conclusion we can make is that the finite line has become an infinite line, clearly a contradiction.

Euclid goes on to say:

"Suppose this done, and let there be left AG less than E."

Now clearly if AG is less than 'E', then AG is a left over, and 'E' is not the unit measure. The process of depletion is not complete. Euclid has arbitrarily stopped the process to justify his 'proof'. We know this because he did not arrive at 'E' by means of depletion. Euclid has committed the unpardonable sin of separating E from the process of depletion, from the whole.

We will now put the proposition in the right perspective.

Referring to the sketch below:
b leaves leftover c
c leaves leftover d
d leaves leftover e
e leaves no leftover
In this case the whole is depleted with the common divisor e which is the unit measure.
The ratio of the part to the whole is 11/28


Note:

a) The leftover diminishes until there is no left over.
b) The leftover becomes the divisor until that which comes  before it, is depleted.
c) The last divisor is the common divisor and establishes the ratio between the whole and the part(s).
d) In an irrational system the part always measures the whole.
e) In a rational system the whole always measures the part.
f ) Note:The sketch is not drawn to scale.

Addendum 5/2005

 To show that incommensurability is a fallacy

Let A B be an extension of finite length x units, then  1/x is the smallest possible unit extension.

       A ------------------------------------------------------------------- B
                                                x units(unity)

Let C cut A B at any position, into two finite sections,
A C and   C B

Let  A C be y/x units so that C B = x/x - y/x = x-y/x units.

      A ---------------------------------------|---------------------------- B
                          y/x                        C          x-y/x

Therefore      A C + C B =  y/x + x-y/x  =  x/x  (unity)

If  y = 1 then the  ratios of A C and C B are:

                                       1/x and x-1/x  respectively

If  y > 1 and y < x then the ratios are:

                                       y/x and x-y/x respectively

It is clear that y cannot be less than 1(smallest part) or more than x(unity).

Therefore A C and C B cannot be incommensurable with A B.
                                      
Note:
The common divisor 1/x is established by means of the depletion process as outlined in " The process of depletion."

A practical example of depletion.(For the infidel teacher)                                      
                                X units total length

         |--------------------------------------|--------------------------|
        A                      X1 units        C           X2 units     B

Assume the depletion process takes place as follows:

     X - X1 = X2                             X1 + X2 = X
     X1 -(2)X2 = X3                    (2)X2 +X3 = X1
     X2 - X3 = X4                           X3 + X4 = X2
     X3 - (5)X4 = X5                   (5)X4 +X5 = X3
     X4 - X5 =  X6                          X5 + X6 = X4
     X5 - X6 = (3)X6(Depleted)   X6+(3)X6 = X5

The ratios are determined as follows:

            X6 = 1
            X5 = 4
            X4 = 5
            X3 = 29
            X2 = 34
            X1 = 97
            X   = 131

Addendum 15/6/2013

Not only is incommensurability a fallacy, what is more, is that real numbers are not real, they are irrational numbers in the true sense of the word, there is no place for them in science and mathematics.